Mathematics with Bubbles

This activity has been developed by Dr Tudur Davies and Prof. Simon Cox, lecturers at the Department of Mathematics, Aberystwyth University.

There are two versions of the activity, the first aimed at learners in years 10-11 and the second at learners in years 12-13, although anybody can have a go at the exercises!
Click on the version of the activity that you want to have a go at below before continuing.
Contact information
Please feel free to contact us with any queries or comments about this activity by emailing maths@aber.ac.uk .
Mathemateg gyda Swigod

Mae'r gweithgaredd hwn wedi'i ddatblygu gan Dr Tudur Davies a'r Athro Simon Cox o Adran Mathemateg, Prifysgol Aberystwyth.

Cefnogir ein darpariaeth cyfrwng Cymraeg gan y Coleg Cymraeg Cenedlaethol.

Mae yna ddau fersiwn o'r gweithgaredd, y naill wedi'i anelu at ddysgwyr ym mlynyddoedd 10-11 a'r llall at ddysgwyr ym mlynyddoedd 12-13. Er hynny, gall unrhyw un roi cynnig ar yr ymarferion!
Cliciwch ar y fersiwn o'r gweithgaredd sy'n fwyaf perthnasol i chi cyn mynd ymlaen.
Manylion cyswllt
Cysylltwch â ni os oes gennych unrhyw gwestiynau neu sylwadau am y gweithgaredd hwn trwy ebostio maths@aber.ac.uk .
Consider a bubble ...
Bubbles have fascinated children for centuries and continue to do so. They're also of interest to some adults! For example, their structure and geometry are of interest to researchers in the mathematical sciences, including some of us at Aberystwyth University. Let's consider the geometry or shape of bubbles ...
Foam research in space!
Liquid foams appear in our day to day lives, for example in frothy coffees, aerated chocolate bars, ice creams, and many personal hygiene and cosmetic products. They are also used in industry, for example to extract oil from rocks or to separate minerals from waste material. Research into foam is conducted so that we better understand how they flow and change over time, with the aim of improving some of the aforementioned products and processes. Some research into foam has even been conducted in space! The image above of floating bubbles has been taken from an experiment conducted on the ESA international space station. More information is given about this research in this youtube video by the ESA.
Have you ever considered why a bubble is spherical? To answer this question mathematically, we might consider the following problem for 2D shapes.
Exercise 1
- Calculate the area of the following regular polygons, which all have a perimeter of 1.
- Calculate the radius and area of a circle with a circumference of 1.
- Calculate the difference between the area of the circle and the area of each polygon considered above. What happens to this difference as the number of sides for the polygon increases?
- What do we conclude from this exercise? Is there another shape with a perimeter of 1 that has a larger area than the circle?


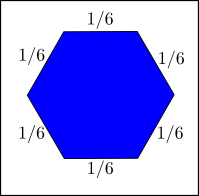

Hint: You might want to partition some of the above into simpler shapes (such as triangles and rectangles) [1].
As we increase the number of sides for a regular polygon (but keep the perimeter fixed at 1) the shape becomes more and more similar to a circle (with circumference 1).
Exercise 1
- Calculate the area of the following regular polygons, which all have a perimeter of 1.
- Show that the area \(A\) of a polygon with \(n\) sides and a fixed perimeter of 1 can be written as the function $$ A(n) = \frac{1}{4n\tan\left(\pi/n\right)}. $$ Hint: Partition a polygon with \(n\) sides into \(n\) identical triangles. [1]
- Show that \(A(n)\) is an increasing function of \(n\).
- Show that $$ \lim_{n\to\infty} A(n) = \frac{1}{4\pi}. $$ Hint: You might want to first make the substitution \(x=1/n\) so that the limit becomes $$ \lim_{n\to\infty}\left(\frac{1}{4n\tan\left(\pi/n\right)}\right)=\lim_{x\to 0} \left(\frac{x}{4\tan\left(\pi x\right)}\right). $$ To determine the value of such a limit, we might be able to substitute in the value that \(x\) approaches (i.e. zero) into our expression and work out the value directly. However, in this example this will give an indeterminate value of 0/0. You might therefore want to try to use the following well-known property: $$ \lim_{x\to 0} \left(\frac{\sin x}{x}\right)=1. $$




Does this mean that we can make the area of our shape (which has a fixed perimeter of 1) as large as we want? The answer to this question is clearly “no”. So what is the largest possible value for the area of a shape which has a perimeter of 1? To calculate this, we need to find the limit of \(A(n)\) as \(n\) tends to infinity.
What do you conclude from the above exercise?
1. Partitioning into triangles is a powerful tool in mathematics for using a computer to understand difficult problems. For example, foam researchers at Aberystwyth use the Surface Evolver software to generate approximations of 3D foams structures using triangulated surfaces.↩
Ystyriwch swigen ...
Mae swigod wedi hudo plant ers canrifoedd ac maent yn parhau i wneud hynny. Maent hefyd o ddiddordeb i rai oedolion! Er enghraifft, mae eu strwythur a'u geometreg o ddiddordeb i ymchwilwyr yn y gwyddorau mathemategol, gan gynnwys rhai ohonom yma'n Mhrifysgol Aberystwyth. Gadewch i ni ystyried geometreg neu siâp swigod ...
Ymchwil ewyn yn y gofod!
Mae ewynnau hylif yn ymddangos yn gyson yn ein bywydau bob dydd, er enghraifft mewn pethau fel coffi ffrothiog, bar siocled, hufen iâ, a nifer o gynnyrch hylendid personol a chosmetig. Maent hefyd yn cael eu defnyddio mewn diwydiant, er enghraifft i echdynnu olew o greigiau neu i wahanu mwynau o ddeunydd gwastraff. Mae gwaith ymchwil i ewyn yn cael ei wneud er mwyn i ni fedru deall yn well sut maent yn llifo ac yn newid dros amser, gyda'r nod o wella rhai o'r cynnyrch a'r prosesau a soniwyd amdanynt. Mae gwaith ymchwil diweddar i ewyn wedi cymryd lle yn y gofod! Mae'r ddelwedd uchod o swigod yn arnofio wedi'i gymryd o arbrawf a gafodd ei wneud ar orsaf ofod rhyngwladol yr ESA. Cewch fwy o wybodaeth am y gwaith ymchwil hwn trwy wylio'r fideo youtube yma gan yr ESA.
Ydych chi erioed wedi ystyried pam fod swigen yn sfferig? Er mwyn ateb y cwestiwn hwn yn fathemategol, efallai ei bod yn werth i ni ystyried yr ymarfer canlynol ar gyfer siapiau 2D.
Ymarfer 1
- Cyfrifwch arwynebedd y polygonau rheolaidd canlynol, sydd i gyd â pherimedr o 1.
- Cyfrifwch radiws ac arwynebedd cylch gyda cylchedd o 1.
- Cyfrifwch y gwahaniaeth rhwng arwynebedd y cylch ac arwynebedd pob un o'r polygonau uchod. Beth sy'n digwydd i'r gwahaniaeth hwn wrth i ni gynyddu'r nifer o ymylon sydd gan y polygon?
- Beth yw eich casgliad ar ôl cwblhau'r ymarfer hwn? Oes yna unrhyw siâp arall gyda pherimedr o 1 sydd ag arwynebedd mwy na'r cylch?




Awgrym: Efallai y byddwch angen rhannu rhai o'r siapiau uchod i siapiau symlach (fel trionglau a phetryalau) [1].
Wrth i ni gynyddu'r nifer o ymylon sydd gan ein polygon rheolaidd (tra'n cadw'r perimedr yn gyson gyda gwerth o 1) mae'r siâp yn dod yn debycach i gylch (gyda cylchedd o 1).
Ymarfer 1
- Cyfrifwch arwynebedd y polygonau rheolaidd canlynol, sydd i gyd â pherimedr o 1.
- Dangoswch y gellir ysgrifennu arwynebedd (\(A\)) polygon rheolaidd gydag \(n\) ymyl, a pherimedr gosodedig o 1, fel y ffwythiant $$ A(n) = \frac{1}{4n\tan\left(\pi/n\right)}. $$ Awgrym: Rhannwch bolygon gydag \(n\) ymyl yn \(n\) triongl unfath. [1]
- Dangoswch fod \(A(n)\) yn ffwythiant cynyddol o \(n\).
- Dangoswch fod $$ \lim_{n\to\infty} A(n) = \frac{1}{4\pi}. $$ Awgrym: Efallai y byddwch yn gyntaf angen gwneud yr amnewidiad \(x=1/n\) fel bod y terfan yn dod i $$ \lim_{n\to\infty}\left(\frac{1}{4n\tan\left(\pi/n\right)}\right)=\lim_{x\to 0} \left(\frac{x}{4\tan\left(\pi x\right)}\right). $$ Er mwyn darganfod gwerth terfannau, mae weithiau'n bosib i amnewid y gwerth mae \(x\) yn dynesu tuag ato (hynny yw sero yn yr enghraifft uchod) i'r mynegiad, a gweithio allan y gwerth yn uniongyrchol. Ond yn yr enghraifft uchod, mae hyn yn rhoi gwerth amhenderfynedig o 0/0. Felly mae'n bosib y byddwch angen ceisio defnyddio'r priodwedd adnabyddus a defnyddiol canlynol rywsut: $$ \lim_{x\to 0} \left(\frac{\sin x}{x}\right)=1. $$




Ydyw hyn yn golygu y gallwn wneud arwynebedd ein siâp (sydd â pherimedr o 1) mor fawr ag yr ydym yn dymuno? Mae'n amlwg mai'r ateb i'r cwestiwn hwn yw “na”. Felly beth yw'r gwerth mwyaf posib ar gyfer arwynebedd siâp sydd â pherimedr o 1? Er mwyn cyfrifo hyn, rydym angen darganfod y terfan o \(A(n)\) wrth i \(n\) dueddu tuag at anfeidredd.
Beth yw eich casgliad o gwblhau'r ymarfer uchod?
1. Mae rhannu siapiau i drionglau yn ddull pwerus mewn mathemateg ar gyfer defnyddio cyfrifiadur i ddatrys a deall problemau anodd. Er enghraifft, mae ymchwilwyr ewyn yn Aberystwyth yn defnyddio meddalwedd y Surface Evolver er mwyn creu brasamcanion o strwythurau ewyn 3D trwy ddefnyddio arwynebau sydd wedi'i creu o drionglau.↩
Minimal Surfaces
In the previous exercise, we saw that in 2D, the circle represents the shape that has the largest area for a given perimeter. Equivalently, we could have shown that the circle is the shape that has the shortest boundary for enclosing a given area. Extending this result to 3D, we can predict that the shape that encloses a given volume with the smallest possible surface area is a sphere. This is slightly more difficult to prove due to the extra spatial dimension, but bubbles confirm that this is the case!
A bubble minimizes its surface area due to surface tension. Therefore might it be possible for us to use bubbles to solve other mathematical problems that require an area or a length to be minimized? By completing this activity, you will have shown that the answer to this question is "yes".
Let's consider a simple example where we'd like to connect two parallel circular rings (as shown below) together with a surface that has the smallest possible area. The connecting surface will touch every point on both rings.
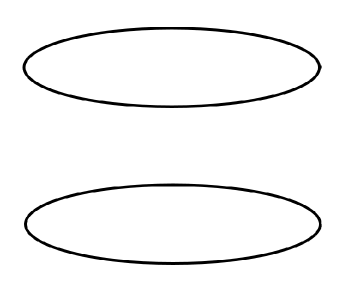
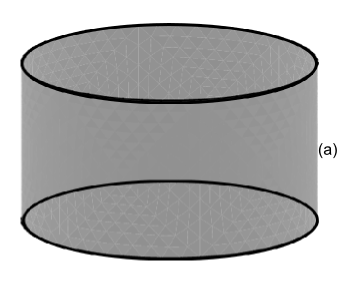
Below are a couple of possible solutions: (a) a cylinder, and (b) a catenoid of the same height. Which one has the smallest surface area?
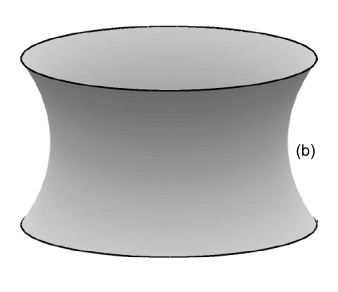
Surprisingly, the catenoid has a smaller surface area than the cylinder. In the video below, two circular wire rings have been dipped in soapy water. The soap film that connects them forms the minimal surface that we want, and it's a catenoid!
More on catenoids
A catenoid is a surface that is generated by rotating a catenary curve around an axis. They are of particular interest to mathematicians due to the special properties that their curvature satisfies (see later). This shape also features in many unexpected and important applications. For example, the liquid bridges that connect particles such as sand grains together have a catenoid shape. So catenoids influence a whole field of research in granular flow!
You may also have noticed that cooling towers in oil refineries or power stations are shaped like a catenoid. This is because the catenoid shape accelerates the airflow inside the towers. Catenoids also offer more stiffness or strength compared to a cylinder of the same height, which is maybe why they’re so often seen in architectural structures.
Curvature
Let's consider a surface that is defined by the graph of the function \(z=f(x,y)\). The curvature of a surface describes how bent or curved it is, and is something that generally varies along the surface. At each point on a surface, there are two "principal" radii of curvature, denoted by \(R_1\) and \(R_2\), as shown in the diagram below.
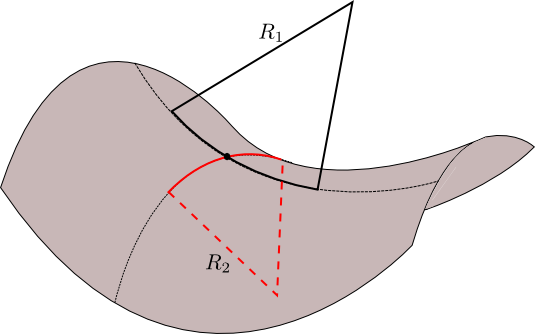
Roughly speaking, \(R_1\) and \(R_2\) denote the radius of the circles that can be fitted onto two particular curves on the surface that are perpendicular to each other at the point of interest. These two curves capture the maximum and minimum bending of the surface at this point. The mean curvature at this point is $$ H = \frac{1}{2}\left(\frac{1}{R_1}+\frac{1}{R_2}\right). $$ A flat plane has zero curvature, so you can think of \(R_1\) and \(R_2\) being infinite in this case. Let's practice finding the principal radii for some familiar shapes ...
Exercise 2
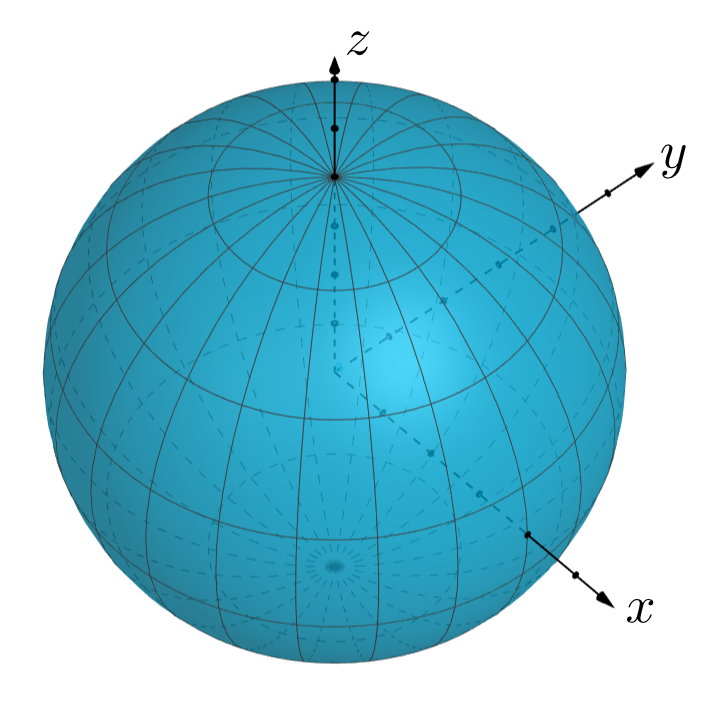
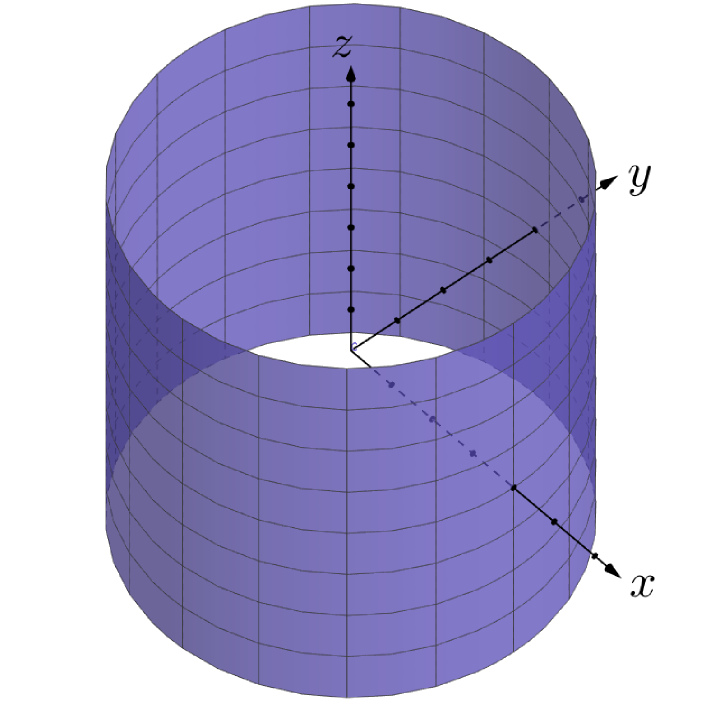
Find the principal radii of curvature, \(R_1\) and \(R_2\), for a
- sphere with radius \(r=4\), and
- a cyllinder with radius \(r=4\) (and some height \(h\)).
As a further exercise, consider the torus that is generated by revolving a circle with radius \(r=2\) and centre \((4,0)\) around the vertical (\(z\)) axis as shown below.
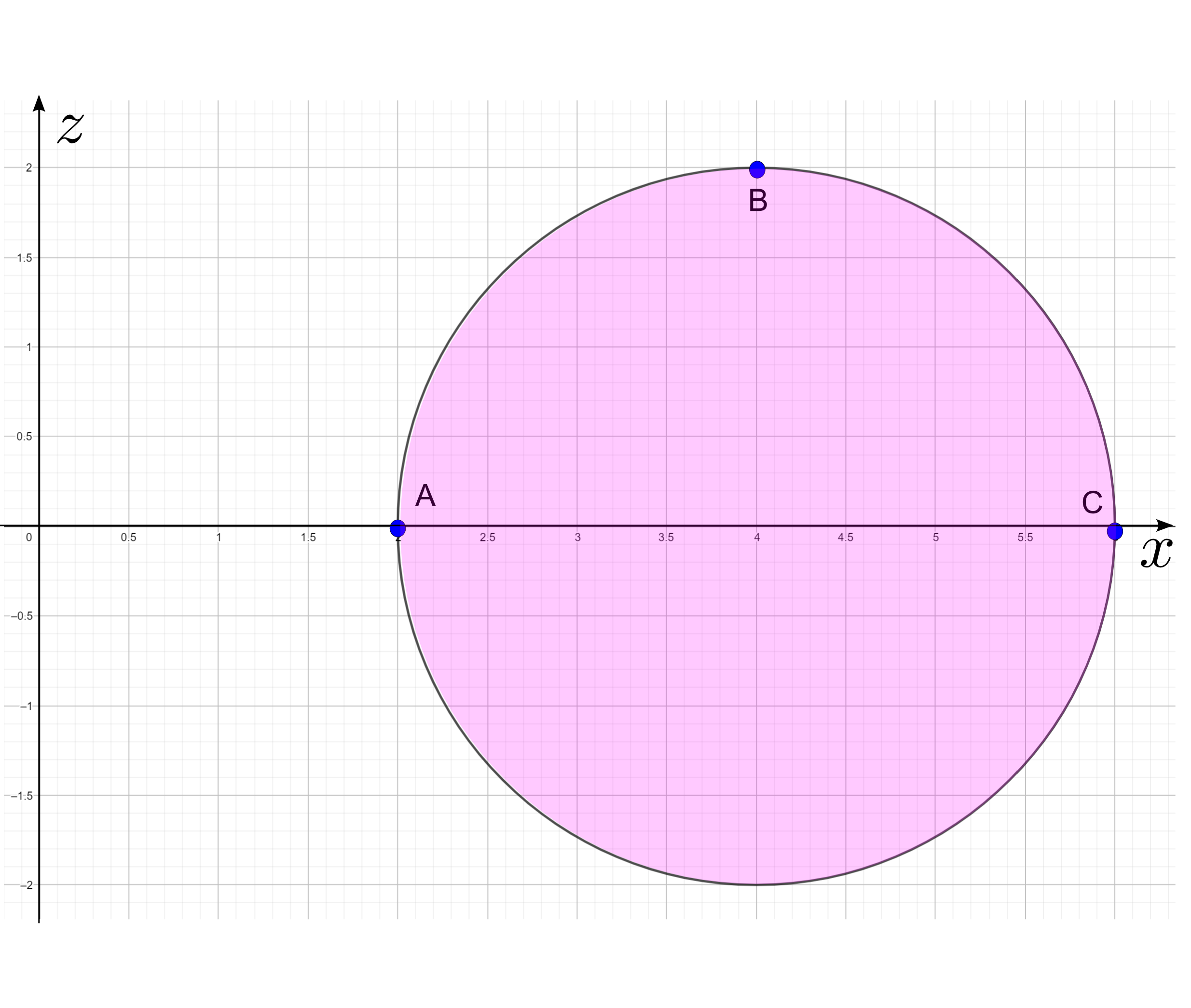
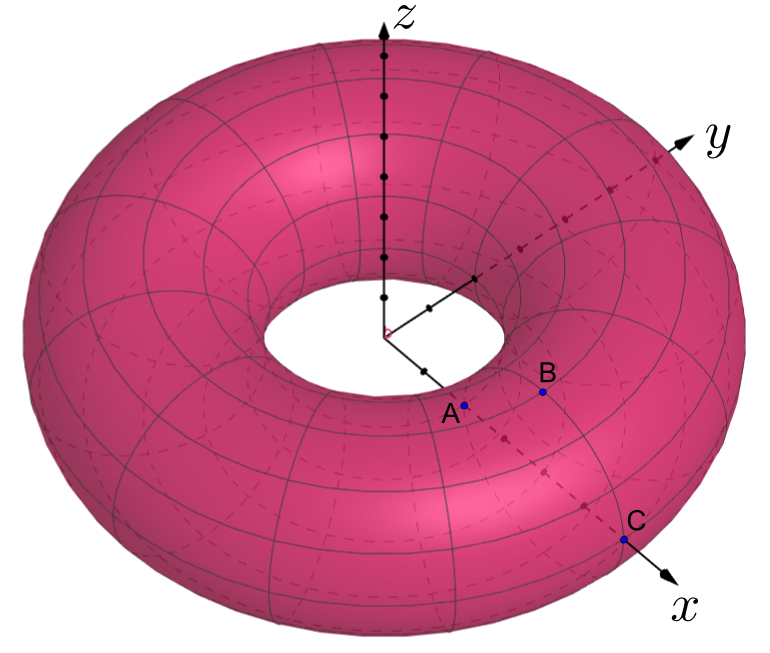
Find the values of the principal radii of curvature, \(R_1\) and \(R_2\), for the surface of this torus at the points (i) A \((2,0,0)\), (ii) B \((4,0,2)\) and (iii) C \((6,0,0)\) (as labelled in the diagrams above).
Exercise 2
Find the principal radii of curvature, \(R_1\) and \(R_2\), for a
- sphere with radius \(r=4\), and
- a cyllinder with radius \(r=4\) (and some height \(h\)).


As a further exercise, consider the torus that is generated by revolving a circle with radius \(r=2\) and centre \((4,0)\) around the vertical (\(z\)) axis, as shown below.


Find the values of the principal radii of curvature, \(R_1\) and \(R_2\), for the surface of this torus at the points (i) A \((2,0,0)\), (ii) B \((4,0,2)\) and (iii) C \((6,0,0)\), as labelled in the diagrams above.
For a soap film, the mean curvature \(H\) is constant. A minimal surface (such as a catenoid) has the special property that the constant mean curvature is zero, that is $$ \frac{1}{2}\left(\frac{1}{R_1}+\frac{1}{R_2}\right)=0. $$ Our aim is to find surfaces that satisfy the above equation. However this is a difficult problem, and not many known solutions exist. So we will use soap films to find such solutions!
For a soap film, the mean curvature \(H\) is constant. A minimal surface (such as the catenoid) has the special property that the constant mean curvature is zero everywhere, that is $$ \frac{1}{2}\left(\frac{1}{R_1}+\frac{1}{R_2}\right)=0. $$ Our aim is to find surfaces that satisfy this equation, so let us briefly delve into the mathematics that this would involve before we continue with the activity.
To find a minimal surface mathematically, we might first introduce the variables \(u\) and \(v\) and parameterize the equation \(z=f(x,y)\) to something like $$ x(u,v) = (u,v,f(u,v)). $$ Determining the curvature of a surface at a point will require evaluating some first and second derivatives of the function \(f\). Because \(f\) is a function of two variables, these will be partial derivatives. In fact, a minimal surface satisfies the following partial differential equation $$ \left(1+f_v^2\right)f_{uu}-2f_uf_vf_{uv}+\left(1+f_u^2\right)f_{vv}=0, $$ where \(f_v=\frac{\partial f}{\partial v}\), \(f_{uv}=\frac{\partial^2 f}{\partial u\partial u}\) etc. At this stage, you might be relieved to hear that solving this partial differential equation is beyond the scope of this activity! The techniques required would be taught at university level mathematics. In fact, very few solutions to this equation are known, and so it's an active area of research to understand them and to find more. In this activity, we're going to use soap films to find such solutions!
Arwynebau Minimol
Yn yr ymarfer blaenorol, fe ddangoson ni mai'r cylch yw'r siâp 2D sydd gyda'r arwynebedd mwyaf ar gyfer perimedr penodol. Yn gyfatebol, gallem fod wedi dangos mai'r cylch yw'r siâp sydd gyda'r ffin byrraf ar gyfer amgau arwynebedd penodol. O ymestyn y canlyniad hwn i 3D, gallwn ragdybio mai'r siâp sy'n amgau cyfaint penodol gyda'r arwynebedd arwyneb lleiaf posib yw'r sffêr. Mae hyn ychydig yn anoddach i'w brofi oherwydd y dimensiwn gofod ychwanegol, ond mae swigod yn cadarnhau bod y rhagdybiad yn gywir!
Mae swigen yn lleiafsymio ei arwynebedd arwyneb yn naturiol o ganlyniad i rym tensiwn arwyneb. Felly ydyw'n bosib i ni ddenyddio swigod i ddatrys problemau mathemategol eraill lle mae angen lleiafsymio rhyw arwynebedd neu hyd? Trwy gwblhau'r gweithgaredd hwn, fe fyddwch wedi dangos mai'r ateb i'r cwestiwn yma yw "ydi".
Gadewch i ni ystyried enghraifft syml lle rydym am gysylltu dau gylch paralel (fel y dangosir isod) gyda'i gilydd gan ddefnyddio arwyneb sydd gyda'r arwynebedd lleiaf posib. Bydd yr arwyneb sy'n cysylltu'r ddau gylch yn cyffwrdd gyda phob pwynt ar y ddau gylch.

Mae'r diagramau isod yn cynnig dau ddatrysiad posib: (a) silindr, a (b) catenoid o'r un taldra. Pa un sydd gan yr arwynebedd arwyneb lleiaf?


Yn annisgwyl efallai, mae arwynebedd arwyneb y catenoid yn llai nag arwynebedd y silindr. Yn y fideo isod, caiff dwy weiren siâp cylch eu trochi mewn dŵr sebonllyd. Mae'r ffilm sebon sy'n eu cysylltu yn ffurfio'r arwyneb minimol yr ydym ei angen, ac mae'n siâp catenoid!
Mwy ar gatenoidau
Mae catenoid yn arwyneb a gaiff ei greu trwy gylchdroi cromlin catena o gwmpas echelin. Maent o ddiddordeb i fathemategwyr yn bennaf am eu priodweddau arbennig sy'n ymwneud â'u crymedd (gweler nes ymlaen). Mae'r siâp hefyd yn amlwg mewn meysydd annisgwyl a phwysig. Er enghraifft, mae pontydd hylif sy'n cysylltu gronynnau (e.e. rhai tywod) gyda'i gilydd yn gwneud hynny gyda siâp catenoid. Felly mae catenoidau yn dylanwadu ar faes ymchwil llawn mewn llif gronynnog!
Efallai eich bod hefyd wedi sylwi fod tyrau oeri mewn purfeydd olew neu orsafoedd pŵer â siâp fel catenoid. Y rheswm pennaf am hyn yw fod y siâp catenoid yn cyflymu llif yr aer tu mewn i'r tyrau. Mae catenoidau hefyd yn cynnig mwy o anhyblygedd a chryfder o'i gymharu â silindr o'r un taldra, sydd efallai'n cynnig rheswm dros pam eu bod i'w gweld mor gyson mewn strwythurau pensaernïol.
Crymedd
Gadewch i ni ystyried arwyneb sydd wedi'i ddiffinio gan graff y ffwythiant \(z=f(x,y)\). Mae crymedd arwyneb yn disgrfio pa mor grwm ydyw, ac mae'n rhywbeth sydd fel arfer yn amrywio ar hyd yr arwyneb. Ymhob pwynt ar yr arwyneb, mae yna ddau "brif" radiws crymedd, wedi'u dynodi gan \(R_1\) a \(R_2\), fel a ddangosir yn y diagram isod.

Yn fras, mae \(R_1\) a \(R_2\) yn dynodi radiysau'r cylchoedd y gellir eu ffitio ar ddwy gromlin benodol ar yr arwyneb, sy'n berpendicwlar i'w gilydd yn y pwynt dan sylw. Mae'r ddwy gromlin yn dal crymedd mwyaf a lleiaf yr arwyneb yn y pwynt hwn. Y crymedd cymedrig yn y pwynt yw $$ H = \frac{1}{2}\left(\frac{1}{R_1}+\frac{1}{R_2}\right). $$ Mae gan blân gwastad grymedd o sero ymhobman, ac felly gallwch feddwl fod \(R_1\) ac \(R_2\) yn anfeidrol yn yr achos hwnnw. Gadewch i ni ymarfer darganfod y prif radiysau crymedd ar gyfer rhai siapiau cyfarwydd ...
Ymarfer 2
Darganfyddwch y prif radiysau crymedd, \(R_1\) a \(R_2\), ar gyfer
- sffêr gyda radiws \(r=4\), a
- silindr gyda radiws \(r=4\) (a rhyw daldra \(h\)).


Fel ymarfer pellach, ystyriwch y torws a greir trwy gylchdroi cylch â radiws \(r=2\) a chanol yn \((4,0)\) o gwmpas yr echeling fertigol (\(z\)) fel a ddangosir isod.


Darganfyddwch werthoedd y prif radiysau crymedd, \(R_1\) a \(R_2\), ar gyfer arwyneb y torws hwn yn y pwyntiau (i) A \((2,0,0)\), (ii) B \((4,0,2)\) a (iii) C \((6,0,0)\), fel sydd wedi'u labelu ar y diagramau uchod.
Ymarfer 2
Darganfyddwch y prif radiysau crymedd, \(R_1\) a \(R_2\), ar gyfer
- sffêr gyda radiws \(r=4\), a
- silindr gyda radiws \(r=4\) (a rhyw daldra \(h\)).


Fel ymarfer pellach, ystyriwch y torws a greir trwy gylchdroi cylch â radiws \(r=2\) a chanol yn \((4,0)\) o gwmpas yr echeling fertigol (\(z\)) fel a ddangosir isod.


Darganfyddwch werthoedd y prif radiysau crymedd, \(R_1\) a \(R_2\), ar gyfer arwyneb y torws hwn yn y pwyntiau (i) A \((2,0,0)\), (ii) B \((4,0,2)\) a (iii) C \((6,0,0)\), fel sydd wedi'u labelu ar y diagramau uchod.
Ar gyfer ffilm sebon, mae'r crymedd cymedrig \(H\) yn gyson. Mae gan arwyneb minimol (fel y catenoid) y priodwedd arbennig o fod â chrymedd cymedrig cyson o sero, hynny yw $$ \frac{1}{2}\left(\frac{1}{R_1}+\frac{1}{R_2}\right)=0. $$ Ein nod yw darganfod arwynebau sy'n bodloni'r hafaliad uchod. Ond mae hon yn broblem anodd, a dim ond ychydig iawn o ddatrysiadau hysbys sy'n bodoli. Felly byddwn ni'n defnyddio ffilmiau sebon i ddarganfod rhai o'r datrysiadau!
Ar gyfer ffilm sebon, mae'r crymedd cymedrig \(H\) yn gyson. Mae gan arwyneb minimol (fel y catenoid) y priodwedd arbennig o gael crymedd cymedrig cyson o sero ymhobman, hynny yw $$ \frac{1}{2}\left(\frac{1}{R_1}+\frac{1}{R_2}\right)=0. $$ Ein nod yw dargangod arwynebau sy'n bodloni'r hafaliad uchod, ac felly gadewch i ni ystyried y mathemateg fyddai rhywun ei angen er mwyn gwneud hynny, cyn parhau gyda'r gweithgaredd.
Er mwyn darganfod arwyneb minimol yn fathemategol, efallai y buasem yn dechrau trwy gyflwyno'r newidynnau \(u\) a \(v\) a paramedru'r hafaliad \(z=f(x,y)\) i rywbeth fel $$ x(u,v) = (u,v,f(u,v)). $$ Bydd darganfod crymedd rhyw arwyneb mewn pwynt penodol yn gofyn am enrhifo rhai deilliadau cyntaf ac ail ddeilliadau o'r ffwythiant \(f\). Gan fod \(f\) yn ffwythiant o ddau newidyn, bydd y rhain yn ddeilliadau rhannol. Mewn gwirionedd, mae arwyneb minimol yn bodloni'r hafaliad differol rhannol canlynol $$ \left(1+f_v^2\right)f_{uu}-2f_uf_vf_{uv}+\left(1+f_u^2\right)f_{vv}=0, $$ lle mae \(f_v=\frac{\partial f}{\partial v}\), \(f_{uv}=\frac{\partial^2 f}{\partial u\partial u}\) ac yn y blaen. Efallai y byddwch yn falch o glywed fod datrys yr hafaliad differol rhannol hwn y tu hwnt i ffiniau'r gweithgaredd yma! Fe fyddai'r technegau sydd eu hangen yn cael eu dysgu mewn mathemateg lefel prifysgol. Mewn gwirionedd, dim ond ychydig iawn o ddatrysiadau i'r hafaliad sy'n hysbys, ac mae darganfod mwy o ddatrysiadau a'u deall yn faes ymchwil gweithredol. Yn y gweithgaredd hwn, fe fyddwn yn defnyddio ffilmiau sebon i ddarganfod rhai o'r datrysiadau.

Plateau's Laws
Joseph Plateau (1801-1883) was a famous Belgian physicist and mathematician. His research included a careful study of how bubbles fit together. To make this as simple as possible, he dipped wire frames in soap solution so that just a few soap films were formed. He noticed that the following rules were always obeyed by the soap films:
- Soap films only ever meet three at a time; the lines that they meet along show as white lines in the videos below; and the angle between any two soap films is always \(120^\circ\).
- No more or less than four of these lines ever meet at a point (which we call a vertex); they meet at an angle of \(109^\circ\).
Advanced mathematics has shown that these laws of Plateau are true of all minimal surfaces, even though he could only infer this from his experiments.
Wire frame experiment
Let's repeat some of Plateau's experiments by dipping wire frames in soap solution ...
1. Tetrahedral Frame
In the above video, we see that soap films connect the tetrahedral frame together with a structure that satisfies Plateau’s rules. Three surfaces meet at equal angles of \(120^\circ\) at each of the white lines seen in the video. There are four of these lines in total, and they meet each other at equal angles of \(109^\circ\) at a single vertex in the middle of the structure. Notice that the lines are straight and the surfaces are planar.
2. Triangular Prism Frame
For a triangular prism, soap films again connect the frame together so that each line is a meeting point for exactly three surfaces (that meet at equal angles of \(120^\circ\)). These lines meet in fours at equal angles of \(109^\circ\) at two vertices. As before, the lines are straight and the surfaces are planar.
3. Cubic Frame
The surface with the smallest area that connects a cubic frame together includes a small square at the centre of the structure. To ensure that Plateau’s rules are satisfied, the lines seen in this video are slightly curved (so that they meet each other in fours at vertices at angles of \(109^\circ\)). The surfaces themselves remain planar. As before, each line seen in the structure represents a meeting point of exactly three surfaces, and these lines meet in fours at the four vertices of the square.
Do it yourself
You can also check that these rules are satisfied by blowing two bubbles so that they come into contact. Count the number of surfaces that meet at the line generated by the two-bubble stucture. Do the surfaces meet at equal angles of \(120^\circ\)?

Alternatively you could create your own foam using standard washing-up liquid and water!
Plateau's laws in current research
We still use Plateau's laws today, for example to predict the arrangements of bubbles with the least area (see the article by Cox and Flikkema in Elec. J. Combinatorics).
2. Image by Albert Callisto, distributed under a CC BY-SA 4.0 license.↩

Deddfau Plateau
Roedd Joseph Plateau (1801-1883) yn ffisegydd a mathemategydd enwog o Wlad Belg. Roedd ei ymchwil yn cynnwys astudiaeth fanwl o sut mae swigod yn ffitio at ei gilydd. Er mwyn gwneud hyn mor syml â phosib, fe drochodd fframiau gwifren mewn hydoddiant sebon fel mai dim ond ychydig o ffilmiau gaiff eu ffurfio. Fe sylwodd fod y ffilmiau sebon bob amser yn bodloni'r rheolau canlynol:
- Mae ffilmiau sebon bob amser yn cwrdd fesul tri; mae'r llinellau lle maent yn cwrdd yn ymddangos fel llinellau gwyn yn y fideos isod; ac mae'r ongl rhwng unrhyw ddau ffilm sebon bob amser yn \(120^\circ\).
- Mae'r llinellau'n cyfarfod mewn pwynt (yr ydym yn ei alw'n fertig) fesul pedwar bob tro; maent yn cyfarfod ar ongl o \(109^\circ\).
Mae mathemateg datblygiedig wedi dangos fod y deddfau uchod yn wir ar gyfer pob arwyneb minimol, er mai dim ond casglu hyn o'i arbrofion a wnaeth Plateau.
Arbrawf ffram gwifren
Gadewch i ni ailadrodd rhai o arbrofion Plateau trwy drochi fframiau gwifren mewn dŵr sebonllyd ...
1. Ffrâm Tetrahedrol
Yn y fideo uchod, rydym yn gweld fod y ffilmiau sebon yn cysylltu'r ffrâm tetrahedrol gyda'i gilydd â strwythur sy'n bodloni deddfau Plateau. Mae tri arwyneb yn cwrdd gydag onglau cyfartal o \(120^\circ\) ymhob un o'r llinellau gwyn a welir yn y fideo. Mae yma bedair llinell wen i gyd, ac mae'r rhain yn cyfarfod gydag onglau cyfartal o \(109^\circ\) mewn un fertig yng nghanol y strwythur. Sylwch fod y llinellau'n syth a'r arwynebau'n blanar.
2. Ffrâm Prism Trionglog
Ar gyfer prism trionglog, mae'r ffilmiau sebon eto'n cysylltu'r ffrâm gyda'i gilydd fel bod pob llinell yn fan cyfarfod i dri yn union o arwynebau (sy'n cwrdd ag onglau cyfartal o \(120^\circ\)). Mae'r llinellau yma'n cyfarfod fesul pedwar gydag onglau cyfartal o \(109^\circ\) mewn dwy fertig. Fel yn yr enghraifft flaenorol, mae'r llinellau'n syth a'r arwynebau'n blanar.
3. Ffrâm Ciwbig
Mae'r arwyneb â'r arwynebedd lleiaf sy'n cysylltu ffram siâp ciwb gyda'i gilydd yn cynnwys ffilm sebon siâp sgwâr yng nghanol y strwythur. Er mwyn sicrhau fod deddfau Plateau’n cael eu bodloni, mae'r llinellau sydd i'w gweld yn y fideo ychydig yn grwm (fel eu bod yn cyfarfod mewn fertigau fesul pedwar ag onglau cyfartal o \(109^\circ\)). Mae'r arwynebau eu hunain yn parhau i fod yn blanar. Fel ynghynt, mae pob llinell yn y strwythur yn fan cyfarfod i dri arwyneb, a phob fertig yn fan cyfarfod i bedair llinell.
Rhowch gynnig arni eich hun
Gallwch chi wirio os yw deddfau Plateau yn cael eu bodloni gan ffilmiau sebon trwy chwythu dwy swigen fel eu bod yn dod i gyswllt. Cyfrwch y nifer o arwynebau sy'n cyfarfod yn y llinell mewn strwythur dwy swigen. Ydyw'r arwynebau'n cwrdd ag onglau cyfartal o \(120^\circ\)?

Neu gallech greu eich ewyn eich hun gan ddefnyddio hylif golchi safonol a dŵr!
Deddfau Plateau mewn ymchwil cyfredol
Rydym yn parhau i ddefnyddio deddfau Plateau hyd heddiw, er enghraifft i ragdybio'r trefniant swigod sydd â'r arwynebedd lleiaf (gweler yr erthygl gan Cox a Flikkema yn Elec. J. Combinatorics) neu er mwyn ymrannu siapiau 3D gan ddefnyddio'r arwynebedd arwyneb lleiaf (gweler yr erthygl gan Davies, Garratt a Cox yn Gwerddon).
2. Delwedd gan Albert Callisto, wedi ryddhau o dan drwydded CC BY-SA 4.0. ↩
Steiner Problem
The Steiner problem is a problem in combinatorial optimization that is named after Swiss mathematician Jakob Steiner. The problem is stated as follows:
Given n cities on a plain (ignoring rivers etc.), what is the arrangement of roads joining them together so that the total length is minimised?
Previously, we considered the 3D problem of finding a minimal surface for connecting a wire frame. Here, we have a 2D problem in which we’d like to find the minimal length for connecting n point (or cities). These two problems are clearly connected, and our observations from the 3D experiment can therefore help us to understand the 2D problem! We could try to transform our solutions to the 3D problem into 2D, by reducing the surfaces and lines in the 3D solutions to lines and vertices in the 2D problem.
Let's consider the best way of connecting three, four and five cities together by completing the exercises below.
Exercise 3: Three Cities
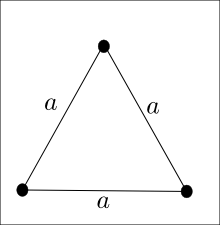
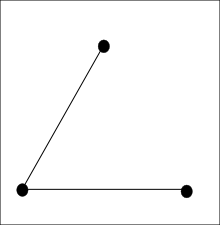
Let's suppose that we have three cities which are conveniently positioned at corners of an equilateral triangle of side-length \(a\).
- Calculate the total length of the roads given in each of the four possible solutions below.
- Which one has the shortest length? Can you improve further on this solution?
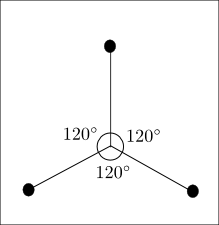
Exercise 3: Three Cities
Let's suppose that we have three cities which are conveniently positioned at corners of an equilateral triangle of side-length \(a\).
- Calculate the total length of the roads given in each of the solutions given below.
-
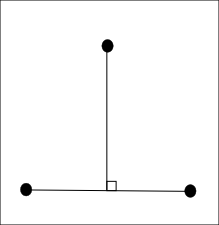
We now suppose that the solution must include exactly one vertex that is positioned inside the triangle. This vertex will be a meeting point for three roads. We expect, from our observations of Plateau’s rules in 3D, that our solution must be symmetric, and therefore we propose that the line between the city in the upper corner of the triangle and the vertex inside the triangle must be vertical. We can vary the position of the vertex along the vertical centreline of the triangle by varying the value of \(\theta\).
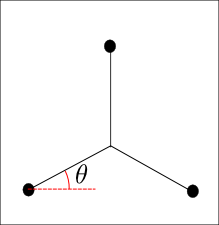
Let \(L(\theta)\) denote the total length of the roads as a function of \(theta\) for this setup. Show that $$ L(\theta) = \frac{a}{2}\left(\frac{2-\sin\theta}{\cos\theta}\right)+\frac{\sqrt{3}}{2}a, $$ for \(\theta\in(0,60^\circ)\).
- Find the absolute minimum value of \(L(\theta)\) for \(\theta\in(0,60^\circ)\). Does this minimum value occur at the value of \(\theta\) that you expected?



We can again use soap films to find the optimal solution. Here we dip two parallel plates that are connected together by three pins that represent the cities.
The optimal solution for connecting three cities includes three lines (i.e. roads) that meet at a vertex at equal angles of \(120^\circ\)!
Exercise 4: Four Cities
We now consider the slightly harder problem for four cities. For this problem, we again arrange our cities conveniently so that they're positioned at the corners of a square with sides of length \(a\). There are many ways in which you can connect these cities together, but which is the shortest?
Maybe you can by now apply what you've learnt about Plateau's laws to predict which one of the solutions below has the shortest length? Calculate the length of each suggested solution. Does the result match your intuition?
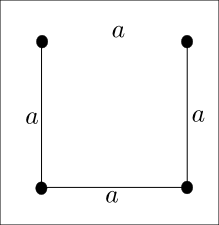
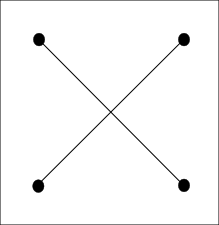


Exercise 4: Four Cities
We now consider the slightly harder problem for four cities. For this problem, we again arrange our cities conveniently so that they're positioned at corners of a square with sides of length \(a\).
- Calculate the total length of the roads given in each of the solutions below. Can you improve on these solutions?
-
Let us now consider solutions that have exactly two vertices that are positioned inside the square. Each vertex will be a meeting point for three roads. There are many possible ways in which the vertices can be positioned, and you can try to generate a few solutions of your own. To ensure that our solution is symmetric, we position the two vertices on the horizontal centre-line of the square (as shown in the diagram below). Note that we could have taken a different convention and positioned the vertices on the vertical centre-line of the square. To ensure symmetry, the angle between the horizontal axis and the roads from the two cities at the bottom of the square both have the same value, which we denote by \(\phi\).
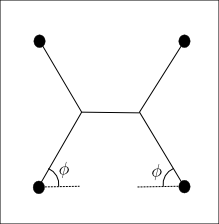
The position of the vertices along the horizontal centreline of the square (and the length of the structure) can be varied by changing the value of \(\phi\). Let \(L(\phi)\) denote the total length of the roads as a function of \(\phi\).
- Find the absolute minimum value of \(L(\phi)\) for \(\phi\in(45^\circ,90^\circ)\). Does this minimum value occur at the value of \(\phi\) that you expected?



Show that $$ L(\phi) = 4\left(\frac{a}{2\sin\phi}\right)+a\left(1-\frac{1}{\tan\phi}\right), $$ where \(\phi\in(45^\circ,90^\circ)\).
We can again use soap films to check our solution.
Exercise 5: Five Cities
In real life, cities will not always be positioned at corners of regular polygons! Let us consider a more realistic problem.
We wish to propose a new transport strategy for the Welsh Government. We wish to connect the five towns and cities from the map below by building the shortest road or railway track possibe.
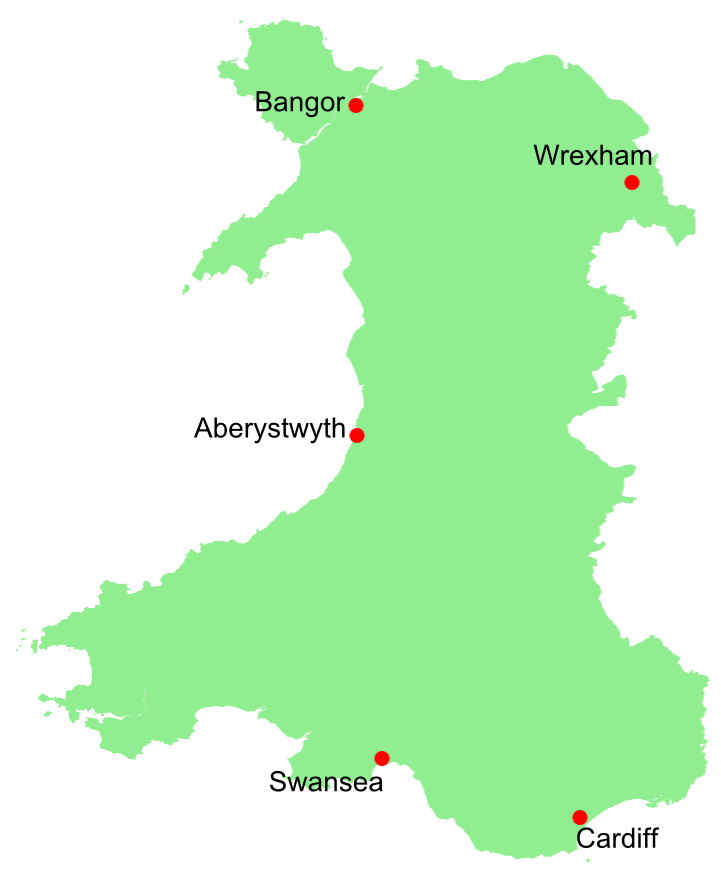
Can you predict what the optimal solution is?
From previous exercises, we know that the best solution will have lines that meet at vertices at equal angles in threes. There are possibly many solutions that satisfy this rule for this irregular arrangement of towns and cities. Here, the trigonometric calculations involved will be harder than for previous examples and determining the total length for each possible solution will take a lot of effort. It may also not be absolutely clear if we’ve considered all possible solutions. This is an example of when a mathematician would turn to a computer to do the tedious work for us.
Alternatively, we can use soap films to solve the problem as in the previous exercises! To see the solution to this problem, please complete our short questionnaire after clicking on "finish" below.
Exercise 5: Five Cities
In real life, cities will not always be positioned at corners of regular polygons! Let us consider a more realistic problem.
We wish to propose a new transport strategy for the Welsh Government. We wish to connect the five towns and cities from the map below by building the shortest road or railway track possibe.

Can you predict what the optimal solution is?
From previous exercises, we know that the best solution will have lines that meet at vertices at equal angles in threes. There are possibly many solutions that satisfy this rule for this irregular arrangement of towns and cities. All but one of these solutions will represent a local minima for the total length and not the absolute minima that we want. Previously, we arranged our cities into corners of regular polygons, so that the calculation of the total length of roads was as simple as possible. Here, the trigonometric calculations involved will be harder and determining the total length for each possible solution will take a lot of effort. It may also not be absolutely clear if we’ve considered all possible solutions. This is an example of when a mathematician would turn to a computer to do the tedious work involved. A computer simulation could easily consider all possible ways in which we can connect the cities together, and it could allow the positions of the three fold vertices to move until an absolute minimum length has been found.
Alternatively, we can use soap films to solve the problem as in the previous exercises! To see the solution to this problem, please complete our short questionnaire after clicking on "finish" below.
Problem Steiner
Mae problem Steiner yn broblem mewn optimeiddiaeth cyfuniadol sydd wedi ei henwi ar ôl y mathemategydd o'r Swistir, Jakob Steiner. Caiff y broblem ei nodi isod:
Ar gyfer \(n\) dinas ar wastadedd (sydd heb unrhyw rwystrau fel afonydd ac yn y blaen), beth yw'r trefniant byrraf o ffyrdd sy'n eu cysylltu?
Yn flaenorol, fe wnaethom ystyried y broblem 3D o ddarganfod arwynebau minimol er mwyn cysylltu fframiau gwifrau. Yn awr, mae gennym broblem 2D lle rydym am ddarganfod yr hyd byrraf ar gyfer cysylltu \(n\) pwynt (neu ddinas). Mae yna gysylltiad amlwg rhwng y ddwy broblem, ac mae'n bosib y bydd ein arsylwadau o'r arbrawf 3D o gymorth i ni ddeall y broblem 2D. Fe allem geisio addasu ein datrysiadau i'r broblem 3D fel eu bod yn berthnasol mewn 2D. Er enghraifft, ydyw'n bosib i ni geisio lleihau'r arwynebau a'r llinellau yn y broblem 3D i linellau a fertigau yn y broblem 2D?
Gadewch i ni ystyried y ffordd orau o gysylltu tair, pedair a phum dinas â'i gilydd trwy gwblhau'r ymarferion isod.
Ymarfer 3: Tair Dinas
Tybiwch fod gennym dair dinas, sydd yn gyfleus wedi cael eu lleoli yng nghorneli triongl hafalochrog gydag ymylon hyd \(a\).
- Cyfrifwch gyfanswm hyd y ffyrdd ymhob un o'r pedwar datrysiad a gynigir isod.
- Pa un sydd â'r hyd byrraf? Fedrwch chi wella ymhellach ar y datrysiad hwn?




Ymarfer 3: Tair Dinas
Tybiwch fod gennym dair dinas, sydd yn gyfleus wedi cael eu lleoli yng nghorneli triongl hafalochrog gydag ymylon hyd \(a\).
- Cyfrifwch gyfanswm hyd y ffyrdd ym mhob un o'r datrysiadau a gynigir isod.
-
Rydym 'nawr yn tybio fod yn rhaid i'r datrysiad gynnwys un fertig yn union, y tu mewn i'r triongl. Bydd y fertig yma'n fan cyfarfod ar gyfer tair ffordd. Rydym yn disgwyl, o'n arsylwadau o reolau Plateau mewn 3D, fod yn rhaid i'n datrysiad fod yn gymesur. Rydym felly'n cynnig fod y llinell rhwng y ddinas sydd wedi ei lleoli yng nghornel uchaf y triongl a'r fertig yn gorfod bod yn fertigol. Gallwn amrywio lleoliad y fertig ar hyd llinell ganol fertigol y triongl trwy amrywio gwerth yr ongl \(\theta\) o'r diagram isod.

Gadewch i \(L(\theta)\) ddynodi cyfanswm hyd y ffyrdd fel ffwythiant o \(\theta\) ar gyfer y strwythur hwn. Dangoswch fod $$ L(\theta) = \frac{a}{2}\left(\frac{2-\sin\theta}{\cos\theta}\right)+\frac{\sqrt{3}}{2}a, $$ ar gyfer \(\theta\in(0,60^\circ)\).
- Darganfyddwch y gwerth lleiaf posib o \(L(\theta)\) ar gyfer \(\theta\in(0,60^\circ)\). Ydyw'r minima yma'n cymryd lle yn y gwerth o \(\theta\) oeddech yn ei ddisgwyl?



Gallwn unwaith eto ddefnyddio ffilmiau sebon er mwyn darganfod y datrysiad optimaidd. Yn yr achos hwn, rydym yn trochi dwy blât baralel sydd wedi'u cysylltu gyda thri pin sy'n cynrychioli'r dinasoedd mewn dŵr sebonllyd.
Mae'r datrysiad optimaidd ar gyfer cysylltu tair dinas yn cynnwys tair llinell (neu ffyrdd) sy'n cyfarfod mewn fertig gydag ongl gyfartal o \(120^\circ\) rhyngddynt.
Ymarfer 4: Pedair Dinas
Gadewch i ni ystyried y broblem ar gyfer pedair dinas. Rydym eto'n trefnu'r dinasoedd yn gyfleus fel eu bod yng nghorneli sgwâr gydag ochrau o hyd \(a\). Mae yna nifer o ffyrdd y gellid cysylltu'r dinasoedd yma gyda'i gilydd, ond pa un sydd â'r hyd byrraf?
Efallai y gallwch erbyn hyn weithredu'r hyn yr ydych wedi'i ddysgu am ddeddfau Plateeau er mwyn rhagdybio pa un o'r datrysiadau isod sydd â'r hyd byrraf? Cyfrifwch gyfanswm yr hyd ar gyfer pob un o'r datrysiadau yma. Ydyw'r canlyniad yn cydfynd â'ch greddf?




Ymarfer 4: Pedair Dinas
Gadewch i ni ystyried y broblem ar gyfer pedair dinas. Rydym eto'n trefnu'r dinasoedd yn gyfleus fel eu bod yng nghorneli sgwâr gydag ochrau o hyd \(a\). Mae yna nifer o ffyrdd y gellid cysylltu'r dinasoedd yma gyda'i gilydd, ond pa un sydd â'r hyd byrraf?
- Cyfrifwch gyfanswm hyd y ffyrdd ar gyfer pob un o'r datrysiadau isod. Fedrwch chi wella ar y datrysiadau yma?
-
Gadewch i ni ystyried datrysiadau sydd â dwy fertig yn union y tu mewn i'r sgwâr. Bydd pob fertig yn fan cyfarfod ar gyfer tair ffordd. Mae yna nifer o drefniadau posib ar gyfer y ffyrdd yn yr achos yma, a gallwch roi cynnig ar greu rhai ohonynt eich hun.
Er mwyn sicrhau fod y datrysiad yn gymesur, rydym yn penderfynu gosod y ddwy fertig ar linell ganol llorweddol y sgwâr (fel a ddangosir yn y diagram isod). Noder y buasem wedi gallu dewis gosod y fertigau ar linell ganol fertigol y sgwâr (a buasem yn cael yr un datrysiad yn y pendraw). Er mwyn sicrhau cymesuredd, mae'r ongl (\(\phi\)) rhwng yr echelin llorweddol a'r ffyrdd o'r dinasoedd yng nghorneli isaf y sgwâr yr un fath ar gyfer y ddwy ddinas.

Rydym yn amrywio lleoliad y fertigau ar hyd llinell ganol llorweddol y sgwâr trwy amrywio gwerth \(\phi\). Trwy wneud hynny, bydd hyd y llinell llorweddol sy'n cysylltu'r ddwy fertig yn amrywio (a bydd y datrysiad yn parhau i fod yn gymesur o gwmpas llinell ganol fertigol y sgwâr). Gadewch i \(L(\phi)\) ddynodi cyfanswm hyd y ffyrdd fel ffwythiant o \(\phi\).
- Darganfyddwch y gwerth lleiaf posib o \(L(\phi)\) ar gyfer \(\phi\in(45^\circ,90^\circ)\). Ydyw'r minima hwn yn cymryd lle yn y gwerth o \(\phi\) yr oeddech yn ei ddisgwyl?



Dangoswch fod $$ L(\phi) = 4\left(\frac{a}{2\sin\phi}\right)+a\left(1-\frac{1}{\tan\phi}\right), $$ ar gyfer \(\phi\in(45^\circ,90^\circ)\).
Rydym eto'n defnyddio ffilmiau sebon i wirio'n datrysiad.
Ymarfer 5: Pum dinas
Mewn bywyd go iawn, nid yw dinasoedd bob amser wedi'u trefnu fel eu bod yn gorwedd yng nghorneli polygonau rheolaidd! Gadewch i ni ystyried problem mwy realistig.
Rydym yn dymuno cynnig strategaeth drafnidiaeth newydd i Lywodraeth Cymru. Rydym yn dymuno cysylltu'r pum tref neu dinas isod trwy adeiladu'r ffordd neu'r rheilffordd fyrraf.
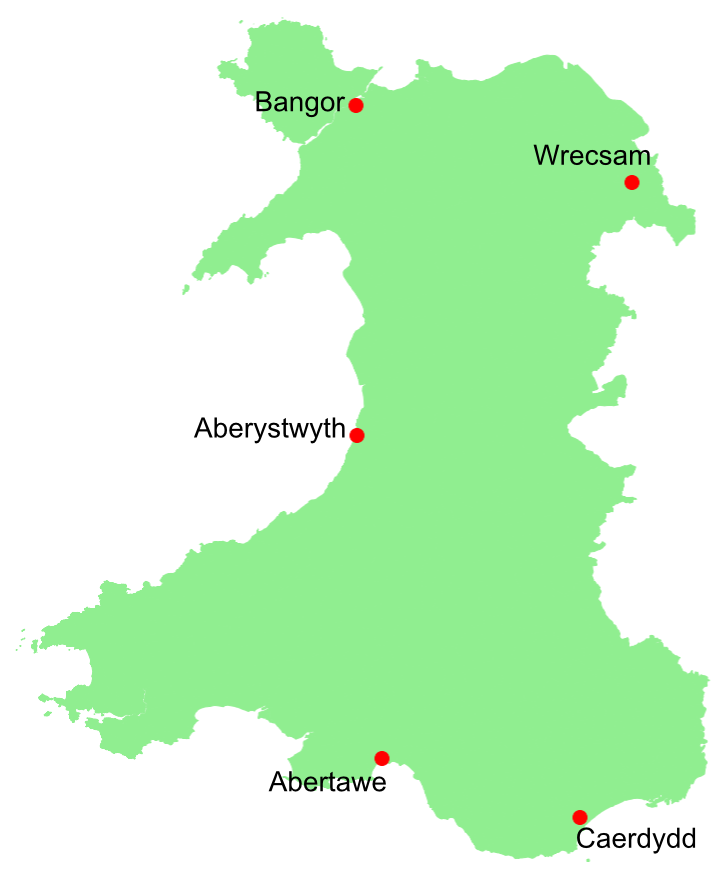
Ydych chi'n gallu rhagdybio'r datrysiad optimaidd yn yr achos hwn?
Fe wyddom o'r ymarferion blaenorol ein bod yn chwilio am ddatrysiad sy'n cynnwys ffyrdd sy'n cyfarfod mewn fertigau fesul tri ar onglau cyfartal i'w gilydd bob tro. Mae'n bosib fod yna nifer o ddatrysiadau sy'n bodloni'r rheol yma ar gyfer y trefniant afreolaidd o drefi a dinasoedd. Fe fydd y cyfrifiadau trigonometrig yn fwy cymleth nag ar gyfer yr enghreifftiau blaenorol ac felly bydd cyfrifo cyfanswm hyd y ffyrdd ar gyfer pob datrysiad yn golygu tipyn mwy o waith. Mae'n bosib hefyd na fyddwn yn gwbl sicr ein bod wedi ystyried pob datrysiad posib. Dyma enghraifft lle bydd mathemategydd yn troi tuag at gyfrifiadur fel bod hwnnw'n gwneud y gwaith cyfrifo anniddorol ar ein cyfer.
Fel arall, gallwn eto ddefnyddio ffilmiau sebon er mwyn datrys y broblem! Er mwyn gweld ein datrysiad i'r broblem hon, cwblhewch ein holiadur byr ar ôl clicio ar y botwm "gorffen" isod.
Ymarfer 5: Pum dinas
Mewn bywyd go iawn, nid yw dinasoedd bob amser wedi'u trefnu fel eu bod yn gorwedd yng nghorneli polygonau rheolaidd! Gadewch i ni ystyried problem mwy realistig.
Rydym yn dymuno cynnig strategaeth drafnidiaeth newydd i Lywodraeth Cymru. Rydym yn dymuno cysylltu'r pum tref neu dinas isod trwy adeiladu'r ffordd neu'r rheilffordd fyrraf.

Ydych chi'n gallu rhagdybio'r datrysiad optimaidd yn yr achos hwn?
Fe wyddom o'r ymarferion blaenorol ein bod yn chwilio am ddatrysiad sy'n cynnwys ffyrdd sy'n cyfarfod mewn fertigau fesul tri ar onglau cyfartal i'w gilydd bob tro. Mae'n bosib fod yna nifer o ddatrysiadau sy'n bodloni'r rheol yma ar gyfer y trefniant afreolaidd o drefi a dinasoedd. Bydd pob un heblaw un o'r datrysiadau yma'n dynodi minima lleol yn hytrach na'r minima absoliwt ar gyfer cyfanswm hyd y ffyrdd. Yn yr enghreifftiau blaenorol, fe wnaethom drefnu ein dinasoedd fel eu bod yn gorwedd yng nghorneli polygonau rheolaidd, yn bennaf er mwyn sicrhau fod y cyfrifiadau mor syml â phosib. Fe fydd y cyfrifiadau trigonometrig y tro hwn yn fwy cymleth ac felly bydd cyfrifo cyfanswm hyd y ffyrdd ar gyfer pob datrysiad yn golygu tipyn mwy o waith. Mae'n bosib hefyd na fyddwn yn gwbl sicr ein bod wedi ystyried pob datrysiad posib. Dyma enghraifft lle bydd mathemategydd yn troi tuag at gyfrifiadur fel bod hwnnw'n gwneud y gwaith cyfrifo anniddorol ar ein cyfer. Fe fyddai efelychiad cyfrifiadurol yn gallu ystyried pob cyfuniad posib ar gyfer y ffyrdd o gysylltu'r pum dinas, yn ogystal â chaniatau i leoliadau'r fertigau symud nes bod y datrysiad optimaidd wedi cael ei ddarganfod.
Fel arall, gallwn eto ddefnyddio ffilmiau sebon er mwyn datrys y broblem! Er mwyn gweld ein datrysiad i'r broblem hon, cwblhewch ein holiadur byr ar ôl clicio ar y botwm "gorffen" isod.
Diolch am gwblhau ein gweithgaredd!
Rydym yn gobeithio i chi fwynhau gweithio drwy'r ymarferion a bod ein gweithgaredd wedi eich ysbrydoli i
archwilio i feysydd newydd o Fathemateg.
Er mwyn cael mynediad i'r datrysiadau ac i wirio eich gwaith, gofynnwn i chi gwblhau ein holiadur (byr iawn):
Thank you for completing our activity!
We hope that you enjoyed working through our activity and that it inspired you to explore new areas of Mathematics.
To access the solutions to the exercises and check your work, please complete our (very short) questionnaire: